【研究背景】
随着人类的不断发展,土壤冻融过程对于环境和基础设施的影响越来越受到关注。例如,在冬季道路上行驶,车辆会经历路面结冰和解冻的过程,这会对车辆的稳定性和行驶安全造成影响。此外,冻融过程还会对土壤的物理性质和水文循环产生影响。因此,研究土壤的冻融过程对于我们更好地理解和管理自然环境具有重要意义。
【研究内容】
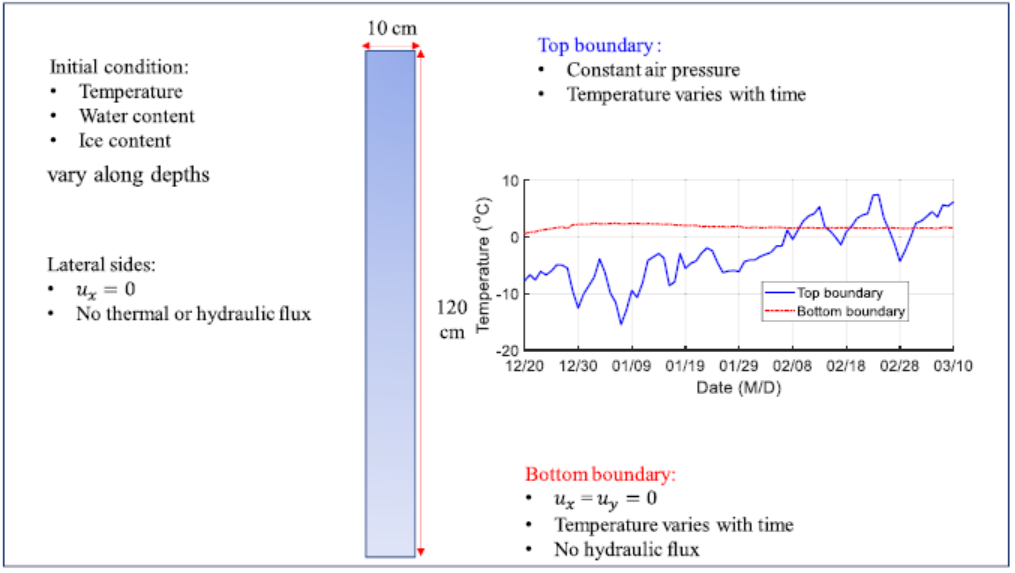
加拿大Concordia大学和南京大学的科研人员合作提出了一种新的热-水-力学模型,用于模拟不饱和土壤中的冻融作用。该模型基于多孔介质连续力学的一般形式推导而来,包含了水分平衡方程和干空气平衡方程。模型考虑了液态水、冰和水蒸气在总水平衡方程中的影响,同时还考虑了非饱和冻结土壤的有效应力定律,以量化孔隙力学行为。该模型还提出了一种基于温度和空气-水毛细压力的非冻结水含量模型。该模型可扩展到包括温暖条件或大变形行为的情况。通过实验室实验数据和在中国西北地区采集的光纤传感数据对该模型进行了验证。
【研究意义】
本研究提出了一种新的热-水-力学模型,用于模拟不饱和土壤中的冻融作用。该模型考虑了多种因素的影响,包括液态水、冰和水蒸气在总水平衡方程中的影响、非饱和冻结土壤的有效应力定律、孔隙力学行为以及非冻结水含量模型等。该模型的创新之处在于它可以用于预测不同条件下土壤的冻融过程,从而有助于我们更好地理解和管理自然地质环境,保护人类生活和生态环境的健康。
来源:Advances in Water Resources
作者:Li B.; Norouzi E.; Zhu H.-H.; Wu B.
出版时间:2024-02-01
DOI:10.1016/j.advwatres.2024.104624
A thermo-poromechanical model for simulating freeze–thaw actions in unsaturated soils
ABSTRACT: This study presents a new fully coupled thermal-hydraulic-mechanical (THM) model for variably saturated freezing soil, which examines the freeze–thaw (F-T) actions. The model is derived based on the general form of continuum mechanics for porous media. The mass balance equations cover the conservations of the total water and dry air, where liquid water, ice, and vapor are involved in the total water balance equation. The effective stress law for the unsaturated frozen soil is included in the model to quantify poromechanical behaviors. The pore pressure contains components from pore water pressure, pore air pressure, and ice pressure. A new model for characterizing the unfrozen water content based on temperature and air-water capillary pressure is proposed. The THM formulation is based on multidimensional derivation, thus is versatile to be extended to cases including warm temperature conditions or large deformation behavior. The model was implemented in a 2D finite element package and validated by a set of published laboratory experimental data. The numerical code is also applied to simulate the freeze–thaw actions in highly unsaturated loess located in the northwest of China, where the quasi-distributed fiber optic sensing data is collected for field-scale validations. Our simulated thermal-hydromechanical responses match well with in situ monitored results and confirm that freezing-induced heaving is still significant in such highly unsaturated soil.
Keywords: Thermo-hydro-mechanical coupling, Unsaturated loess, Finite element method, Pore pressure, Fiber optic sensing
References
Arzanfudi, M.M., Al-Khoury, R., 2018. Freezing-thawing of porous media: an extended finite element approach for soil freezing and thawing. Adv. Water Resour. 119, 210–226.
Bai, R., Lai, Y., Pei, W., Zhang, M., 2020. Investigation on frost heave of saturated–unsaturated soils. Acta Geotech. 15, 3295–3306. https://doi.org/10.1007/s11440-020-00952-6.
Bekele, Y.W., Kyokawa, H., Kvarving, A.M., Kvamsdal, T., Nordal, S., 2017. Isogeometric analysis of THM coupled processes in ground freezing. Comput. Geotech. 88, 129–145.
Cao, D.-F., Zhu, H.-H., Wu, B., Wang, J.-C., Shukla, S.K., 2021. Investigating temperature and moisture profiles of seasonally frozen soil under different land covers using actively heated fiber Bragg grating sensors. Eng. Geol. 290, 106197.
Chen, Y., Lai, Y., Li, H., Pei, W., 2022. Finite element analysis of heat and mass transfer in unsaturated freezing soils: formulation and verification. Comput. Geotech. 149, 104848.
Dall’Amico, M., Endrizzi, S., Gruber, S., Rigon, R., 2011. A robust and energy-conserving model of freezing variably-saturated soil. Cryosph 5, 469–484.
Devoie, ´E.G., Gruber, S., McKenzie, J.M., 2022. A repository of 100+ years of measured soil freezing characteristic curves. Earth Syst. Sci. Data Discuss. 2022, 1–21.
Gawin, D., Pesavento, F., Koniorczyk, M., Schrefler, B.A., 2020. Poro-mechanical model of strain hysteresis due to cyclic water freezing in partially saturated porous media. Int. J. Solids Struct. 206, 322–339.
Gawin, D., Pesavento, F., Koniorczyk, M., Schrefler, B.A., 2019. Non-equilibrium modeling hysteresis of water freezing: ice thawing in partially saturated porous building materials. J. Build. Phys. 43, 61–98.
Ge, S., McKenzie, J., Voss, C., Wu, Q., 2011. Exchange of groundwater and surface-water mediated by permafrost response to seasonal and long term air temperature variation. Geophys. Res. Lett. 38, L14402.
Girgis, N., Li, B., Akhtar, S., Courcelles, B., 2020. Experimental study of rate-dependent uniaxial compressive behaviors of two artificial frozen sandy clay soils. Cold Reg. Sci. Technol. 180, 103166 https://doi.org/10.1016/j.coldregions.2020.103166.
Hansson, K., Sˇimunek, J., Mizoguchi, M., Lundin, L.-C., Van Genuchten, M.T., 2004. Water flow and heat transport in frozen soil: numerical solution and freeze–thaw applications. Vadose Zo. J. 3, 693–704.
Huang, X., Rudolph, D.L., 2021. Coupled model for water, vapour, heat, stress and strain fields in variably saturated freezing soils. Adv. Water Resour. 154, 103945 https://doi.org/10.1016/j.advwatres.2021.103945.
Hughes, T.J.R., 1979. A multidimentional upwind scheme with no crosswind diffusion. Finite Elem. Method. Convect. Domin. Flows 34. AMD.
Jiang, X.-W., Xie, H.-Y., Ge, S., Tang, H., Tan, S.-C., Wang, X.-S., Wan, L., Zeng, Y., 2023. On the extinction depth of freezing-induced groundwater migration. J. Hydrol. 619, 129358 https://doi.org/10.1016/j.jhydrol.2023.129358.
Jun, T.-S., Korsunsky, A.M., 2010. Evaluation of residual stresses and strains using the Eigenstrain Reconstruction Method. Int. J. Solid. Struct. 47, 1678–1686. https://doi.org/10.1016/j.ijsolstr.2010.03.002.
Konrad, J.-M., Morgenstern, N.R., 1980. A mechanistic theory of ice lens formation in fine-grained soils. Can. Geotech. J. 17, 473–486.
Kurylyk, B.L., Watanabe, K., 2013. The mathematical representation of freezing and thawing processes in variably-saturated, non-deformable soils. Adv. Water Resour. 60, 160–177.
Lewis, R.W., Schrefler, B.A., 1998. The Finite Element Method in the Static and Dynamic Deformation and Consolidation of Porous Media. John Wiley & Sons.
Li, B., Akhtar, S., 2022. Characterizations of tensile yield and failure processes of frozen clay soils: laboratory testing and numerical modeling. Bull. Eng. Geol. Environ. 81, 429. https://doi.org/10.1007/s10064-022-02942-2.
Li, P., Li, T., Vanapalli, S.K., 2018. Prediction of soil–water characteristic curve for Malan loess in Loess Plateau of China. J. Cent. South Univ. 25, 432–447.
Li, Z., Chen, J., Tang, A., Sugimoto, M., 2021. A novel model of heat-water-air-stress coupling in unsaturated frozen soil. Int. J. Heat Mass Transf. 175, 121375 https://doi.org/10.1016/j.ijheatmasstransfer.2021.121375.
Ma, F., Liu, E., Song, B., Wang, P., Wang, D., Kang, J., 2022. A poromechanics-based constitutive model for warm frozen soil. Cold Reg. Sci. Technol. 199, 103555.
McKenzie, J.M., Voss, C.I., Siegel, D.I., 2007. Groundwater flow with energy transport and water–ice phase change: numerical simulations, benchmarks, and application to freezing in peat bogs. Adv. Water Resour. 30, 966–983.
Mizoguchi, M., 1990. Water, Heat and Salt Transport in Freezing Soil. Univ. Tokyo, Tokyo. Nishimura, S., Gens, A., Olivella, S., Jardine, R.J., 2009. THM-coupled finite element analysis of frozen soil: formulation and application. Geotechnique 59, 159–171. https://doi.org/10.1680/geot.2009.59.3.159.
Nixon, J.F., 1991. Discrete ice lens theory for frost heave in soils. Can. Geotech. J. 28, 843–859.
Norouzi, E., Li, B., Erkmen, R., 2022a. Modelling equivalent elastic properties of imperfectly bonded soil-rock mixtures using an XFEM-based computational homogenization. Comput. Geotech. 144, 104638 https://doi.org/10.1016/j.compgeo.2022.104638.
Norouzi, E., Li, B., Raymond, J., 2022b. Numerical modeling of thermo-hydromechanical processes related to geothermal heat pump operations in a subarctic climate. In: 56th US Rock Mechanics/Geomechanics Symposium. OnePetro.
Norouzi, E., Moslemzadeh, H., Mohammadi, S., 2019. Maximum entropy based finite element analysis of porous media. Front. Struct. Civ. Eng. 13, 364–379.
O’Neill, K., Miller, R.D., 1985. Exploration of a rigid ice model of frost heave. Water Resour. Res. 21, 281–296.
Painter, S.L., 2011. Three-phase numerical model of water migration in partially frozen geological media: model formulation, validation, and applications. Comput. Geosci. 15, 69–85.
Painter, S.L., Karra, S., 2014. Constitutive model for unfrozen water content in subfreezing unsaturated soils. Vadose Zo. J. 13.
Rempel, A.W., 2007. Formation of ice lenses and frost heave. J. Geophys. Res. Earth Surf. 112.
Rempel, A.W., Wettlaufer, J.S., Worster, M.G., 2004. Premelting dynamics in a continuum model of frost heave. J. Fluid Mech. 498, 227–244. https://doi.org/10.1017/S0022112003006761.
Samimi, S., Pak, A., 2014. A novel three-dimensional element free Galerkin (EFG) code for simulating two-phase fluid flow in porous materials. Eng. Anal. Bound. Elem. 39, 53–63.
Sheshukov, A.Y., Nieber, J.L., 2011. One-dimensional freezing of nonheaving unsaturated soils: model formulation and similarity solution. Water Resour. Res. 47.
Stuurop, J.C., van der Zee, S.E.A.T.M., Voss, C.I., French, H.K., 2021. Simulating water and heat transport with freezing and cryosuction in unsaturated soil: comparing an empirical, semi-empirical and physically-based approach. Adv. Water Resour. 149, 103846.
Thomas, H.R., Cleall, P., Li, Y.-C., Harris, C., Kern-Luetschg, M., 2009. Modelling of cryogenic processes in permafrost and seasonally frozen soils. Geotechnique 59, 173–184.
Van Genuchten, M.T., 1980. A closed-form equation for predicting the hydraulic conductivity of unsaturated soils. Soil Sci. Soc. Am. J. 44, 892–898.
Williams, P.J., Smith, M.W., 1989. The Frozen earth: Fundamentals of Geocryology. Cambridge University Press, Cambridge.
Wu, B., Zhu, H.-H., Cao, D., Xu, L., Shi, B., 2021. Feasibility study on ice content measurement of frozen soil using actively heated FBG sensors. Cold Reg. Sci. Technol. 189, 103332.
Wu, B., Zhu, H.-H., Liu, T.-X., Wang, D.-Y., Hu, L.-L., Li, B., 2023. Experimental investigation of interfacial behavior of fiber optic cables embedded in frozen soil for in-situ deformation monitoring. Measurement 112843. https://doi.org/10.1016/j.measurement.2023.112843.
Yin, X., Lai, Y., Liu, E., 2023. Microstructure-based effective stress for unsaturated frozen soils. Int. J. Numer. Anal. Methods Geomech. 47, 261–274.
Yin, X., Liu, E., Song, B., Zhang, D., 2018. Numerical analysis of coupled liquid water, vapor, stress and heat transport in unsaturated freezing soil. Cold Reg. Sci. Technol. 155, 20–28. https://doi.org/10.1016/j.coldregions.2018.07.008.
Zhang, T., Barry, R.G., Knowles, K., Ling, F., Armstrong, R.L., 2003. Distribution of seasonally and perennially frozen ground in the Northern Hemisphere. In: Proceedings of the 8th International Conference on Permafrost. AA Balkema Publishers, Zürich, Switzerland, pp. 1289–1294.
Zhang, Y., Michalowski, R.L., 2015. Thermal-hydro-mechanical analysis of frost heave and thaw settlement. J. Geotech. Geoenvironm. Eng. 141, 4015027.
Zhou, X., Zhou, J., Kinzelbach, W., Stauffer, F., 2014. Simultaneous measurement of unfrozen water content and ice content in frozen soil using gamma ray attenuation and TDR. Water Resour. Res. 50, 9630–9655. https://doi.org/10.1002/2014WR015640.
Zhu, H.-H., Wu, B., Cao, D.-F., Li, B., Wen, Z., Liu, X.-F., Shi, B., 2023. Characterizing thermo-hydraulic behaviors of seasonally frozen loess via a combined optoelectronic sensing system: field monitoring and assessment. J. Hydrol. 129647 https://doi.org/10.1016/j.jhydrol.2023.129647.
